 |
|
 |
 |
|
 |
. Choosing how many objects: Scroll through the number menus to choose a multiple and a power of ten. For instance, if you choose the number 4 and next to it the number 100,000, the balance will place 400,000 objects on that side of the scale. You will still only see one object, but the scale is weighing 400,000 of them. You can choose fractions, too. If you choose the number 2 and next to it the fraction 1/100, the balance will place 2/100ths of an object on the scale. That is the same as 1/50th of an object.  top
. top
.
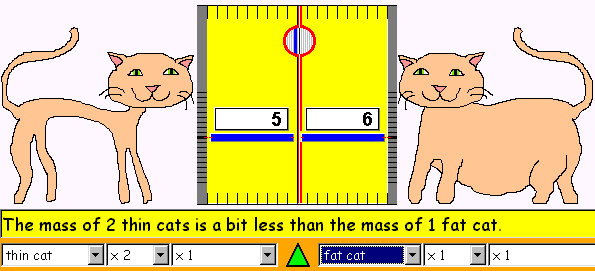
How to balance the objects:
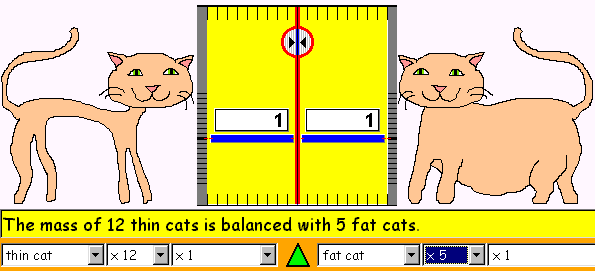 When you are comparing two objects of very different mass, you might multiply one object by a large number and multiply the other side by a fraction.
Not every pairing of objects can be balanced if the difference in their mass is too vast. Even 14 billion electrons do not come close to balancing with one billionth of a thin cat. (When the objects are very far out of balance, the scale will show a gray background. It cannot show all the powers of 10.)
1) Click on the object and a small window will pop up announcing its weight. Choose the unit of measurement you want from the menu. You might find that the actual weights of the objects do not exactly confirm your explorations. Take the example above: we have balanced 12 thin cats with 5 fat cats. If we divide 12 by 5 we get 2.4 ... this means that one fat cat balances with 2.4 thin cats. When we click on each picture to learn their actual weights, what do we find?
  (You may click the menu bar to choose the unit of measurement you like; this example uses kilograms.) If we divide 5 kilograms by 2 kilograms we get 2.5 ... this means that one fat cat balances with 2.5 thin cats. But on the scale, we found that one fat cat balances with 2.4 thin cats. How can this be?
The explanation is that this scale has a tolerance for slight differences in weight. Objects which are almost (but not quite) equal in weight will appear to balance. We've built in this tolerance so that it is easier to find the equilibrium (to get the two sides to balance).
The neutron star is shown through an x-ray telescope. A black hole cannot be viewed directly so we've tried to simulate objects falling into a black hole.
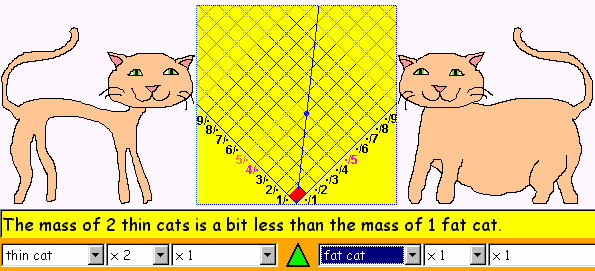 (Compare the rational fulcrum to the "normal" one. When both sides are balanced, the blue line is centered.) . Jumping cats: Explore what happens when you place one or more jumping cats on the scale. Do the jumping cats ever become stable? When? . What is mass? The mass of an object is a measure of the amount of material it contains. An object's mass determines its weight when there is gravity. In a weightless environment an object has no weight - but it always has mass.
____________________ |
Photo Credits
Math Cats Balance © copyright 2003 -
by Wendy Petti of Math Cats & Maurici Carbó Jordi of nummòlt. All rights reserved.